Clinical Evaluation of Drug Effects

General Principles

After pharmacologic agents have stimulated excitable cell-membrane proteins and have produced a physiologic effect, it is important to evaluate the effect clinically. This section focuses on methods of evaluating drug effects such as dose-response curves, efficacy, potency, the median effective dose (ED₅₀ ), the median lethal dose (LD₅₀ ), and the therapeutic index.

Concentration Versus Response Relationships

The standard comparison of a drug with its clinical effect is the concentration versus response curve ( Fig. 3-35 ). The relationship shown is the time-independent relationship between dose, concentration, or some other measure of exposure to the drug (x axis) and the measured effect (y axis). The measured effect can be an absolute response (e.g., twitch height), a normalized response (e.g., percentage of twitch height depression), or a population response (e.g., fraction of subjects moving at incision). The standard equation for this relationship is the Hill equation, sometimes called the sigmoid-E_max relationship:

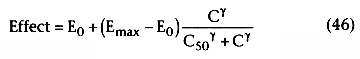
Figure 3-35 Dose or concentration versus response. A sigmoidal concentration-response curve is generated when the log drug concentration is plotted against the clinical response or effect. The concentration-response curve is shifted to the right (B to C) in the presence of a competitive antagonist or desensitization. Curve C may also represent an agonist with a lower receptor affinity (K_a ) or potency than curve B. Curve A represents an agonist with higher receptor affinity or potency than curve B.

Potency and Efficacy

Considerable confusion exists about the definition of potency because of competing definitions of the term. One use of potency is to describe the effect of a given dose of the drug. Consider two different oral preparations of an identical drug: one that dissolves quickly in the stomach and is rapidly absorbed, and one that never completely dissolves and is poorly absorbed. A given dose of the rapidly absorbed formulation can produce a more profound drug effect and therefore be considered more potent than an identical dose of the slowly absorbed formulation. From a therapeutic perspective, potency is often defined in terms of the dose versus response relationship.

From a pharmacologic perspective, potency is best described in terms of the concentration versus response relationship. As shown in Figure 3-35 , a drug with a left-shifted concentration versus response curve (i.e., lower C₅₀ ) is considered more potent, and a right-shifted dose versus response curve renders it less potent. To be precise, potency should be defined in terms of a specific drug effect (e.g., 50% of maximal effect). This is particularly important if the two drugs have different Hill coefficients or efficacies.

Efficacy is a measure of the intrinsic ability of a drug to produce a given physiologic or clinical effect ( Fig. 3-36 ). For example, in G protein-coupled receptors efficacy is influenced by receptor coupling to G proteins, activation of second messengers, and the ability to generate ultimate physiologic responses. The scale used to describe intrinsic efficacy at a given receptor ranges from 0 to 1. Efficacy for full agonists is 1.0, for neutral antagonists is 0, and for partial agonists ranges between 0 and 1.0. In contrast, the term potency refers to the quantity of drug that must be administered to produce a specific effect. Two drugs may have the same efficacy, but if one drug produces the maximum effect at 1 mg and the second drug produces the maximum effect only at 100 mg, the second drug is less potent. The differences between a full agonist and a partial agonist (see Fig. 3-23 and Fig. 3-24 ) represent differences in efficacy.

Effective Dose and Lethal Dose

The ED₅₀ is the dose of a drug required to produce a specific effect in 50% of individuals to whom it is administered. The LD₅₀ is the dose of a drug required to produce death in 50% of patients (or animals) to whom it is administered. The therapeutic index of a drug is the ratio

96

Figure 3-36 Relationships among efficacy, potency, and individual variability as they related to a typical sigmoidal dose or concentration versus response curve.

Drug Interactions

Actions at the Same Receptor

Agonists and antagonists modify dose-response relationships. If two agonists have an identical affinity (K_a ) for a given receptor and identical effectiveness in coupling to second messengers, their resultant dose-response curves should be superimposable. However, if two agonists have different receptor affinities, despite identical effectiveness in coupling to second messengers, their dose-response curves are parallel (with the rightward shifted curve corresponding to the drug with lower receptor affinity) (see Fig. 3-35, curves B and C ). When two agonists are administered simultaneously, the clinical effect depends on the total amount of drug-receptor complexes generated.

Full agonists produce maximal responses, whereas partial agonists produce less than maximal response at the same receptor occupancy. The precise molecular mechanism that explains this blunting of the maximal response by partial agonists is unknown, but it may reflect the ability of full agonists to stabilize R* most effectively. Partial agonists shift the dose-response curve of the agonist to the right (see Fig. 3-35, curves B to C ).

The addition of a competitive antagonist also shifts the dose-response curve to the right (see Fig. 3-35, B to C ). In contrast, the addition of a noncompetitive agonist shifts the dose-response curve to the right and reduces the efficacy of the agonist. This occurs because maximal response cannot be obtained at any agonist dose because blockade of effect (i.e., antagonism) cannot be reversed.

Figure 3-37 Relationships among the median effective dose (ED₅₀ ), median lethal dose (LD₅₀ ), and therapeutic index. These curves were generated from data on animals that were injected with various doses of a sedative-hypnotic and the clinical responses determined. ED₅₀ is the dose of drug required to produce a specific effect (i.e., hypnosis) in 50% of animals to which it is administered (left curve). LD₅₀ is the dose of drug required to produce death in 50% of animals to which drug is administered (right curve). The therapeutic index of the drug is the ratio between LD₅₀ and ED₅₀ (LD₅₀ /ED₅₀ ). LD₁ is the lethal dose in 1% of the population, and ED₉₉ is the effective dose in 99% of the population. As drawn, LD₁ < ED₉₉ , which would not be clinically acceptable.

Actions at Different Receptors

Anesthesia is the practice of applied drug interactions. As discussed in Chapter 31 , general anesthesia is, at a minimum, a combination of hypnosis and analgesia. There has been extensive work looking at the interaction of hypnotic drugs, which include inhaled anesthetics and GABA agonists (i.e., propofol, barbiturates, etomidate, and benzodiazepines), and analgesic drugs, specifically opioids.

A prototypical study of the relationship between inhaled anesthetics and opioids is the investigation by McEwan and colleagues,^[16] who characterized the interaction between isoflurane and fentanyl. The minimum alveolar concentration (MAC) of isoflurane associated with a 50% chance of moving on incision is compared with the plasma fentanyl concentration in Figure 3-38 . Initially, the interaction is profound, with a 50% reduction in isoflurane MAC at a plasma fentanyl concentration of 1.7 ng/mL and a 63% reduction in isoflurane MAC associated with a plasma fentanyl concentration of 3 ng/mL. Beyond 3 ng/mL, there is very little benefit from additional fentanyl. The first clinical implication of this interaction is that a modest amount of opioid dramatically reduces the concentrations of inhaled anesthetic required to prevent movement. The second clinical implication is that some hypnotic component must be added to the anesthetic to prevent movement, even with huge doses of opioids.

97

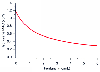Figure 3-38 Influence of fentanyl on the minimum alveolar concentration (MAC) of isoflurane associated with a 50% probability of movement on incision. Modest amounts of fentanyl greatly reduce the MAC of isoflurane, but a ceiling in MAC reduction is quickly reached. Even with large opioid doses, some isoflurane is required to ablate the movement response. (Adapted from McEwan AI, Smith C, Dyar O, et al: Isoflurane minimum alveolar concentration reduction by fentanyl. Anesthesiology 78:864–869, 1993.)

Similar work has been done for propofol. In a series of elegant studies, Vuyk and colleagues^[17] characterized the interaction of propofol with alfentanil. The interaction is markedly synergistic, with modest amounts of alfentanil greatly decreasing the amount of propofol associated with a 50% chance of response to endotracheal intubation or surgical incision ( Fig. 3-39 ). Vuyk and colleagues^[17] also documented the interaction of propofol and alfentanil

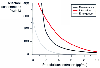Figure 3-39 Influence of alfentanil on the concentration of propofol associated with a 50% probability of response to intubation and incision, as well as a 50% probability of awakening at the end of surgery. Moderate doses of alfentanil greatly reduce the dose of propofol required to ablate the response to noxious stimulation. However, below propofol concentrations of approximately 2 µg/mL, even large doses of alfentanil cannot reliably ablate the response to noxious stimulation. (Adapted from Vuyk J, Lim T, Engbers FH, et al: The pharmacodynamic interaction of propofol and alfentanil during lower abdominal surgery in women. Anesthesiology 83:8–22, 1995.)

Classic interaction studies, such as those previously described, examine the concentrations associated with a particular response (e.g., a 50% chance of moving) for two drugs, evaluated separately and in combination. However, a more general view is that any combination of two drugs is associated with a response. This is best viewed as a response surface in which the x and y axes of the surface are concentrations (or doses) of drugs A and B, and the z axis is the response to the particular combination. Minto and colleagues^[18] proposed a mathematical framework for response surfaces for a variety of interaction surfaces of interest to anesthesiologists. Figure 3-40 shows six examples of response surfaces that apply to a variety of anesthetic drugs.