|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
The specific architectural order of the cardiac muscles provides the basis for the heart to function as a pump. The ellipsoid shape of the left ventricle (LV) is a result of the laminar layering of spiraling bundles of cardiac muscles ( Fig. 18-2 ). The orientation of the muscle bundle is longitudinal in the subepicardial myocardium and circumferential in the middle segment and again becomes longitudinal in the subendocardial myocardium. Because of the ellipsoid shape of the LV, there are regional differences in wall thickness that result in corresponding variations in the cross-sectional radius of the LV chamber. These regional differences may serve to accommodate the variable loading conditions of the LV.[4] In addition, such anatomy allows the LV to eject blood in a corkscrew-type
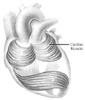 Figure 18-2
Muscle bundles. (From Marieb EN: Human Anatomy
& Physiology, 5th ed. San Francisco, Peason Benjamin Cummings, 2001, p 684.)
Figure 18-2
Muscle bundles. (From Marieb EN: Human Anatomy
& Physiology, 5th ed. San Francisco, Peason Benjamin Cummings, 2001, p 684.)
Unlike the LV, which needs to pump against the higher-pressure systemic circulation, the right ventricle (RV)
 Figure 18-3
Frank-Starling relationship. The relationship between
sarcomere length and tension developed in cardiac muscles is shown. In the heart,
an increase in end-diastolic volume is the equivalent of an increase in myocardial
stretch; therefore, according to Starling's law, increased stroke volume is generated.
Figure 18-3
Frank-Starling relationship. The relationship between
sarcomere length and tension developed in cardiac muscles is shown. In the heart,
an increase in end-diastolic volume is the equivalent of an increase in myocardial
stretch; therefore, according to Starling's law, increased stroke volume is generated.
An intricate matrix of collagen fibers form a scaffold of support for the heart and adjacent vessels. This matrix provides enough strength to resist tensile stretch. The collagen fibers are made up of mostly the thick collagen type I fiber, which cross-links with the thin collagen type III fiber, the other major type of collagen.[5] In close proximity to the collagen fibers are elastic fibers that contain elastin. They account for the elasticity of the myocardium.[6]
The heart provides the driving force to deliver blood throughout the cardiovascular system to supply nutrients and remove metabolic waste. Because of the complexity of RV anatomy, the traditional description of systolic function is usually limited to the left ventricle. Systolic performance of the heart is dependent on loading conditions and contractility. Preload and afterload are two interdependent factors extrinsic to the heart that govern cardiac performance.
Preload is defined as the ventricular load at the end of diastole, before contraction has started. First described by Starling, a linear relationship exists between sarcomere length and myocardial force ( Fig. 18-3 ). In clinical practice, surrogate representatives of left ventricular volume such as pulmonary wedge pressure or central venous pressure are used to estimate preload.[3]
Afterload is defined as systolic load on the LV after contraction has begun. Aortic compliance is an additional
Preload and afterload can be considered as the wall stress that
is present at the end of diastole and during LV ejection, respectively. Wall stress
is a useful concept because it includes preload, afterload, and the energy required
to generate contraction. Wall stress and heart rate are probably the two most relevant
indices that account for changes in myocardial oxygen demand. The law of Laplace
states that wall stress (σ) is the product of pressure (P) and radius (R) divided
by wall thickness (h)[3]
:
σ = P × R/2h
The ellipsoid shape of the LV allows for the least amount of wall stress such that as the ventricle changes its shape from ellipsoid to spherical, wall stress is increased. By using the ratio of the long axis to the short axis as a measure of the ellipsoid shape, a decrease in this ratio would signify a transition from ellipsoid to spherical.
Thickness of the LV muscle is an important modifier of wall stress. For example, in aortic stenosis, afterload is increased. The ventricle has to generate far higher pressure to overcome the increased load opposing systolic ejection of blood. To generate such high performance, the ventricle increases its wall thickness (LV hypertrophy). By applying Laplace's law, increased LV wall thickness will decrease wall stress despite the necessary increase in LV pressure to overcome the aortic stenosis ( Fig. 18-4 ).[7] In a failing heart, the radius of the LV increases, thus increasing wall stress.
The Frank-Starling relationship is an intrinsic property of myocardium by which stretching of the myocardial sarcomere results in enhanced myocardial performance for subsequent contractions (see Fig. 18-3 ). Otto Frank first noted in 1895 that in skeletal muscle, the change in tension was directly related to its length and that in the heart, as pressure changed, a corresponding change in volume occurred.[8] E. H. Starling, using an isolated heart-lung preparation as a model, observed in 1914 that "the mechanical energy set free on passage from the resting to the contracted state is a function of the length of the muscle fiber."[9] If a strip of cardiac muscle is mounted in a muscle chamber under isometric conditions and stimulated at a fixed frequency, an increase in sarcomere length results in an increase in twitch force. Starling concluded that the increased twitch force was the result of a greater interaction of muscle bundles.
Electron microscopy has demonstrated that sarcomere length (2.0 to 2.2 µm) is positively related to the amount of actin and myosin cross-bridging and that there is an optimal sarcomere length at which the interaction is maximal. This concept is based on the assumption that the increase in cross-bridging is equivalent to an increase in muscle performance. Although this theory continues to hold true for skeletal muscle, the force-length relationship in cardiac muscle is more complex. When comparing force-strength relationships between skeletal and cardiac muscle, it is noteworthy that the reduction in force is only 10% even if cardiac muscle is at 80% sarcomere length.[8] The cellular basis of the Frank-Starling mechanism is still being investigated and will be briefly discussed later. A common clinical application of Starling's law is the relationship of left ventricular end-diastolic volume (LVEDV) and stroke volume. The Frank-Starling mechanism may remain intact even in a failing heart.[10] However, ventricular remodeling after injury or in heart failure may modify the Frank-Starling relationship.
Each Frank-Starling curve specifies a level of contractility or the inotropic state of the heart, which is defined as the work performed by cardiac muscle at any given end-diastolic fiber. Factors that modify contractility will create a family of Frank-Starling curves with different contractility ( Fig. 18-5 ).[7] Among the factors that are
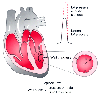 Figure 18-4
In response to aortic stenosis, left ventricular pressure
increases. To maintain wall stress at control levels, compensatory left ventricular
hypertrophy develops. According to Laplace's law, wall stress =
pressure × radius ÷ (2 × wall thickness). Therefore,
the increase in wall thickness offsets the increased pressure and wall stress is
maintained at control levels. (From Opie LH: Ventricular function. In
The Heart. Physiology from Cell to Circulation, 3rd ed. Philadelphia, Lippincott-Raven,
1998, pp 343–389.)
Figure 18-4
In response to aortic stenosis, left ventricular pressure
increases. To maintain wall stress at control levels, compensatory left ventricular
hypertrophy develops. According to Laplace's law, wall stress =
pressure × radius ÷ (2 × wall thickness). Therefore,
the increase in wall thickness offsets the increased pressure and wall stress is
maintained at control levels. (From Opie LH: Ventricular function. In
The Heart. Physiology from Cell to Circulation, 3rd ed. Philadelphia, Lippincott-Raven,
1998, pp 343–389.)
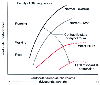 Figure 18-5
A family of Starling curves is shown. A leftward shift
of the curve denotes enhancement of the inotropic state, whereas a rightward shift
denotes decreased inotropy. (From Opie LH: Ventricular function. In
The Heart. Physiology from Cell to Circulation, 3rd ed. Philadelphia, Lippincott-Raven,
1998, pp 343–389.)
Figure 18-5
A family of Starling curves is shown. A leftward shift
of the curve denotes enhancement of the inotropic state, whereas a rightward shift
denotes decreased inotropy. (From Opie LH: Ventricular function. In
The Heart. Physiology from Cell to Circulation, 3rd ed. Philadelphia, Lippincott-Raven,
1998, pp 343–389.)
In isolated muscle, the maximal velocity of contraction, or Vmax,
is defined as the maximal velocity of ejection at zero load. Vmax is obtained by
plotting the velocity of muscle shortening in isolated papillary muscle at varying
degrees of force. Although this relationship can be replicated in isolated myocytes,
Vmax cannot be measured in an intact heart because complete unloading is impossible.
To measure the intrinsic contractile activity of an intact heart, several strategies
have been attempted with varying success. Pressure-volume loops, albeit requiring
catheterization of the left side of the heart, are currently the best way to determine
contractility in an intact heart ( Fig.
18-6
).[7]
The pressure-volume loop represents
an indirect measure of the Starling relationship between force (pressure) and muscle
length (volume). Clinically, the most commonly used noninvasive index of ventricular
contractile function is the ejection fraction, which is assessed by echocardiography,
angiography or radionucleotide ventriculography.
Ejection fraction = (LVEDV − LVESV)/LVEDV,
where LVESV is left ventricular end-systolic volume.
The work of the heart can be divided into external and internal work. External work is expended to eject blood under pressure, whereas internal work is expended within the ventricle to change the shape of the heart and prepare the heart for ejection. Internal work contributes
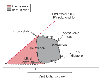 Figure 18-6
Pressure-volume loop. Point a
depicts the start of isovolumic contraction. The aortic valve opens at point b,
and ejection of blood follows (b→c).
The mitral valve opens at d, and ventricular filling
ensues. External work is defined by a, b, c, and
d and internal work by e, d,
and c. The pressure-volume area is the sum of external
and internal work. (From Opie LH: Ventricular function. In
The Heart. Physiology from Cell to Circulation, 3rd ed. Philadelphia, Lippincott-Raven,
1998, pp 343–389.)
Figure 18-6
Pressure-volume loop. Point a
depicts the start of isovolumic contraction. The aortic valve opens at point b,
and ejection of blood follows (b→c).
The mitral valve opens at d, and ventricular filling
ensues. External work is defined by a, b, c, and
d and internal work by e, d,
and c. The pressure-volume area is the sum of external
and internal work. (From Opie LH: Ventricular function. In
The Heart. Physiology from Cell to Circulation, 3rd ed. Philadelphia, Lippincott-Raven,
1998, pp 343–389.)
External work or stroke work is a product of the stroke volume
(SV) and pressure (P) developed during ejection of the stroke volume.
Stroke work = SV × P or (LVEDV − LVESV) ×
P
The external work and internal work of the ventricle both consume O2 . The clinical significance of internal work is illustrated in the case of a poorly drained LV during cardiopulmonary bypass. Although external work is provided by the roller pump during bypass, myocardial ischemia can still occur because poor drainage of the LV creates tension on the LV wall and increases internal work.
The efficiency of cardiac contraction is estimated by the following
formula[12]
:
Cardiac efficiency = External work/Energy equivalent of
oxygen consumption
The corkscrew motion of the heart for the ejection of blood is the most favorable in terms of work efficiency based on the architecture in a normal LV (with the cardiac muscle bundles arranged so that a circumferentially oriented middle layer is sandwiched by longitudinally oriented outer layers). In heart failure, ventricular dilation reduces cardiac efficiency because it increases wall stress, which in term increases oxygen consumption.[11]
In isolated cardiac muscle, an increase in the frequency of stimulation induces an increase in the force of contraction. This relationship is termed the "treppe"
Diastole is ventricular relaxation, and it occurs in four distinct phases: (1) isovolumic relaxation; (2) the rapid filling phase, that is, the LV chamber filling at variable LV pressure; (3) slow filling, or diastasis; and (4) final filling during atrial systole. The isovolumic relaxation phase is energy dependent. During the auxotonic relaxation phases (phases 2 through 4), ventricular filling occurs against pressure. It encompasses a period during which the myocardium is unable to generate force and filling of the ventricular chambers takes place. The isovolumic relaxation phase does not contribute to ventricular filling. The vast amount of ventricular filling occurs in the second phase, whereas the third phase adds only about 5% of the total diastolic volume and the final phase provides 15% of ventricular volume from atrial systole.
To assess diastolic function, several indices have been developed. The most widely used index for examining the isovolumic relaxation phase of diastole is to calculate the peak instantaneous rate of LV pressure decline (-dP/dt) or the time constant of isovolumic LV pressure decline (τ). The aortic closing-mitral opening interval or the isovolumic relaxation time and the peak rate of LV wall thinning as determined by echocardiography have both been used to estimate diastolic function during auxotonic relaxation. Ventricular compliance can be evaluated by pressure-volume relationships to determine function during the auxotonic phases of diastole.[12] [14]
Many different factors influence diastolic function: systolic volume load, passive chamber stiffness, elastic recoil of the ventricle, the diastolic interaction between the two ventricular chambers, atrial properties, and catecholamines. Whereas systolic dysfunction is a reduced ability of the heart to eject, diastolic dysfunction is a decreased ability of the heart to fill. Abnormal diastolic function is now being recognized as the predominant cause of the pathophysiology of congestive heart failure.[15]
Ventricular interactions during systole and diastole are internal mechanisms that function as internal feedback to modulate stroke volume. Systolic ventricular interaction involves the effect of the interventricular septum on the function of both ventricles. Because the interventricular septum is anatomically linked to both ventricles, it will be part of the load against which each ventricle has to work. Therefore, any changes in one ventricle will also be present in the other. In diastolic ventricular interaction, dilatation of either the LV or RV will have an impact on effective filling of the contralateral ventricle and thereby modify function.
|
|
|
|
|
|
|
|
|
|
|
|
|